Fractal Structure of Microcrystalline Cellulose Obtained by Method of Spray Drying-Juniper Publishers
JUNIPER
PUBLISHERS- ACADEMIC JOURNAL OF POLYMER SCIENCE
Abstract
In this research, a fractal structure of beads of
different sizes obtained by spray-drying of aqueous dispersions of
microcrystalline cellulose (MCC) was studied. These beads are formed as a
result of aggregation of rod-shaped cellulose nanocrystalline particles
(CNP). It was found that increasing the average radius (R) of the
formed MCC beads results in increased porosity (P) and reduced density
(ρ). The dependences of P and ρ on scale factor (R/r) can be expressed
by power-law equations: p=(R/r)k-dp and p-d (R/r)DP-K
,where the fractal dimensions PD= 2.887 andPD=2.986 are close to
Euclidean dimension E=3 for three-dimensional space; r=3nm is radius of
cellulose nanocrystalline particles, Po = 0.03cm3/g is porosity and
d=1.585g/cm3 is true density (specific gravity) of CNP, respectively.
Thus, with the increase in the size of formed MCC beads, the order in
the packing of the beads is distorted conforming to theory of
diffusion-limited aggregation process.
Keywords: Microcrystalline cellulose (MCC); Spray-drying; MCC bead; Size; Porosity; density; Fractal dimension
Introduction
It is known that various natural and artificial
objects and phenomena can be considered as fractals, distinctive
features of which are scale invariance (self-similarity) and fractional
dimension [1,2]. The theory of fractals is widely used in engineering,
mathematics, biology, physics, chemistry and other areas. According to
this theory, the fractal dimension (D) of an object can be determined by
logarithmization of power-law dependence of structure or property on
scale factor. In particular, the theory of fractals was applied for
description of structure and properties of such wide-spread natural
biopolymer as cellulose and also of diverse cellulose materials.
For instance, cellulose fibers were studied by a
method of low-temperature nitrogen sorption to measure the dependence of
cumulative volume on radius of various pores expressed by the power-law
function, from which the fractal dimension from 2.88 to 2.95 was
determined [3]. In another research [4], the fractal structure of pores
in various cellulose materials was studied by nitrogen and water vapor
sorption methods; in the case of nitrogen sorption the fractal dimension
of pores was from 2.13 to 2.50, whereas sorption of water vapor gave
the fractal dimension of pores less than 1.5 due to altering of
cellulose structure under effect of water. The study the distribution of
nano-scale cellulose aggregates by method of small-angle X-ray
scattering permitted to calculate the fractal dimension D=2.10 [5].
The main purpose of this research was to study the
fractal characteristics of Microcrystalline Cellulose (MCC) beads with
different sizes prepared by method of spray drying, which can be used as
excipient in pharmaceutics and medicine, as well as filler in various
composite materials.
Experimental
Material
The initial material was chemical grade cotton cellulose (99 % α-cellulose, DP=2700) of Hercules Inc.
Method of preparation beads of MCC
Cotton cellulose was hydrolyzed with boiling 1.5M
sulfuric acid at the acid/cellulose ratio 10 for 1h followed by
filtration of the acid and washing of MCC on the filter to neutral pH
[6]. The resulting wet cake of MCC was diluted with distilled water to
obtain 1-5% dispersions, which were disintegrated in Waring blender at
15,000rpm for 10min to break the agglomerates. To produce beads, the
aqueous dispersions of MCC were spray-dried using a lab drier of
Pilotech at the following conditions: feeding 10ml/min, air pressure
0.2MPa, inlet temperature 120oC and outlet temperature 60oC.
Sieve analysis

MCC beads of different sizes were separated by
screening through sieves with mesh of 80-100, 140-170, 230-270 and
450-635. The average radius of the beads was shown in the Table 1. With
rise of the concentration of MCC dispersion from 1 to 5%, an increase in
the yield of larger beads was observed.
Scanning electron microscopy
Shape of MCC beads were investigated by electron microscope
Hitachi S-4700.
Wide-angle X-ray scattering (WAXS)
Nanostructure of MCC beads was investigated by WAXS
method using Rigaku-Ultima Plus diffractometer (CuKa - radiation,
l=0.15418nm) [7, 8]. Lateral size (L) of cellulose nanocrystalline
particles (CNP) in direction perpendicularly to [200] planes of
crystalline unit cell were calculated by modified Scherrer equation
taking into account the contribution of instrumental factor and
lattice distortions to width of crystalline peak. Minimum radius of
CNP was calculated as follows: r = 0.5L.
Sorption method
Sorption of hexane vapor by MCC beads was measured at 25oC
with the use of a vacuum Mac-Ben apparatus having helical spring
quartz scales [9]. The porosity [p,cm3/g] the beads was calculated
by the equation:
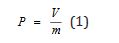
where V is total volume of pores (cm3 ) measured at relative
vapor pressurep/p0 m is mass of the dry sample (g).
Results and Discussion
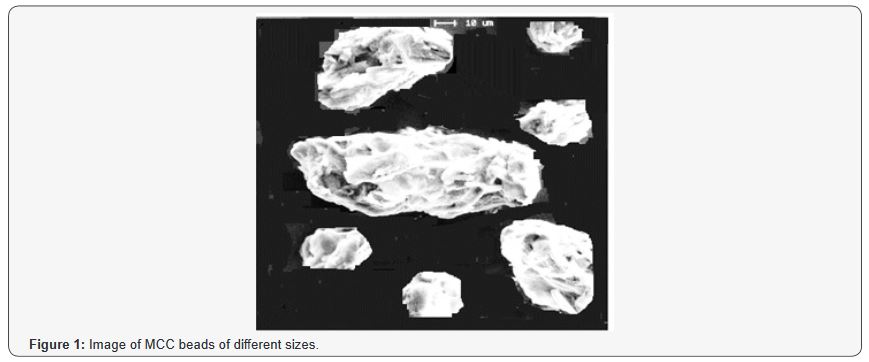
SEM studies showed that spray-dried MCC beads have
ellipsoidal or spherical shape and contain small rod-like
particles (Figure 1). As known, cellulose is semicrystalline
linear polysaccharide that consists of elementary nanofibrils
and their bundles called microfibrils [10]. Furthermore, each
nano-scale fibril is built of ordered rod-like nanocrystallites and
low ordered amorphous nanodomains. The three-dimensional
ordered nanocrystallites are strong and inaccessible structural
elements. As against, the low-ordered amorphous domains are
weak and accessible segments of the fibrils. Therefore, cleavage of
amorphous domains at the acid hydrolysis leads to formation of
rod-shaped nanocrystalline particles (CNP). As a result of spraydrying,
the rod-shaped CNP are aggregated by their side surfaces
and forms micron sized beads of microcrystalline cellulose with
various average radius.
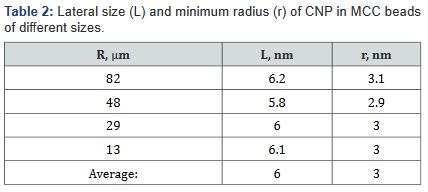
The structural studies showed that CNP isolated from plant
biomass have lateral sizes of 4-8nm and length of 100-200nm
[11]. As it follows from WAXS results, the average lateral size (L)
of CNP made of cotton cellulose is 6nm and their average radius
(r) is 3nm or 0.003μm (Table 2).
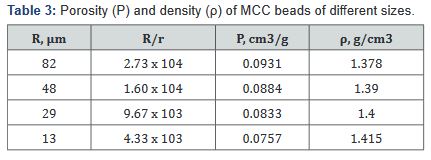
The study of vapor sorption of inert organic liquid (hexane)
revealed that the porosity of MCC beads varies in the range from
0.0757 to 0.0931 cm3/g (Table 3). Moreover, when the average size
of the beads increases, their porosity rises.
The dependence of porosity (P) on scale factor (R/r) can be
expressed by a power-law function:
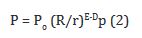
where Po is porosity of CNP, DP is fractal dimension; E=3 is
Euclidean dimension for three-dimensional space.
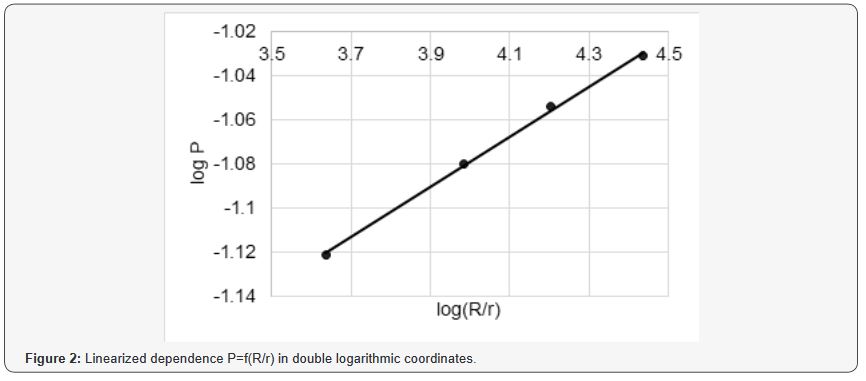
After logarithmizing of the function (2), a linear graph was
drawn (Figure 2), from which the values of Po = 0.03 [cm3/g]=2.887 were found.
Along with porosity, it is also possible to calculate the density
of MCC beads, as follows:
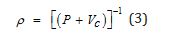
where Vc = d-1 is specific volume of CNP having crystallinity
about 80% and true density (specific gravity) d=1.585g/cm3 [10].
Using ρ values and scale factor [R/r](Table 3), the linear
dependence can be obtained (Figure 3) by logarithmizing of the
function:
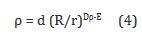
From the linearized graph (Figure 3), the value of Dρ =2.986
was calculated.
The results have shown that the small beads contain more
densely packed aggregates of CNP than the large MCC beads.
According to DLA-theory [2, 12-14], such structural feature
indicates that the formation of MCC beads occurs via diffusionlimited
aggregation process.
Conclusion
Rod-shaped crystalline nanoparticles (CNP) of cotton cellulose
with radius r = 3 nm have a compact packing with high true density
(specific gravity) d=1.585g/cm3 and negligible porosity Po=0.03
cm3/g During spray-drying a lateral aggregation of rod-shaped CNP
occurs. Moreover, with the development of aggregation process of
CNP and increase in size of the formed MCC beads, the order in
the packing is distorted conforming to theory of diffusion-limited
aggregation process. Consequence of this phenomenon is increase
in porosity and decrease in density with the rise in size (average
radius R) of the beads. The dependences of porosity (P) and
density (ρ) on scale factor (R/r) can be expressed by the following
power-law equations:
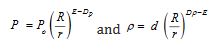
where the fractal dimensions DP = 2.887 and Dρ = 2.986 are
close to Euclidean dimension E=3 for three-dimensional space.
For more articles in Academic Journal of Polymer
Science please click on:
https://juniperpublishers.com/ajop/index.php
https://juniperpublishers.com/ajop/index.php

Comments
Post a Comment